Bohr’s theory on the model of the hydrogen atom (1913) has made an important contribution to the knowledge of the behavior of atoms; however, its theory is not able to give a complete description of the behavior of electrons and has been evident since from its formulation that additional factor must exist.
In 1926, Erwin Schrödinger elaborated an equation that describes the behavior and energy of sub-atomic particles in general, an equation analogous to Newton’s laws of motion in classical physics.
The Schrödinger equation can predict both the corpuscular behavior in terms of the particle’s mass m and the wave behavior in terms of a wave function ψ, which describes the system’s position in space (e.g., the position of an electron in an atom).
The wave function has no direct physical meaning. However, its square is proportional to the probability of finding the particle in a particular region in space.
In practice, the most likely place to find a particle is where the wave function’s value is highest.
A consequence of this principle is that in an electron orbiting in an atom, we cannot speak of a trajectory but a region of space. The probability of finding the electron is different from zero.
Quantum Mechanical Description of the Atom
This ‘quantum mechanical’ approach to the atom requires that the notion of an orbit for an electron be somehow overcome, speaking instead of an orbital, which can be thought of as the wave function of an electron in an atom.
When an electron is said to be in a specific orbital, the distribution of electron density is described by the wave function associated with that orbital. To be precise, the probability of locating the electron in space is given by the value of the square of the wave function at a given point.
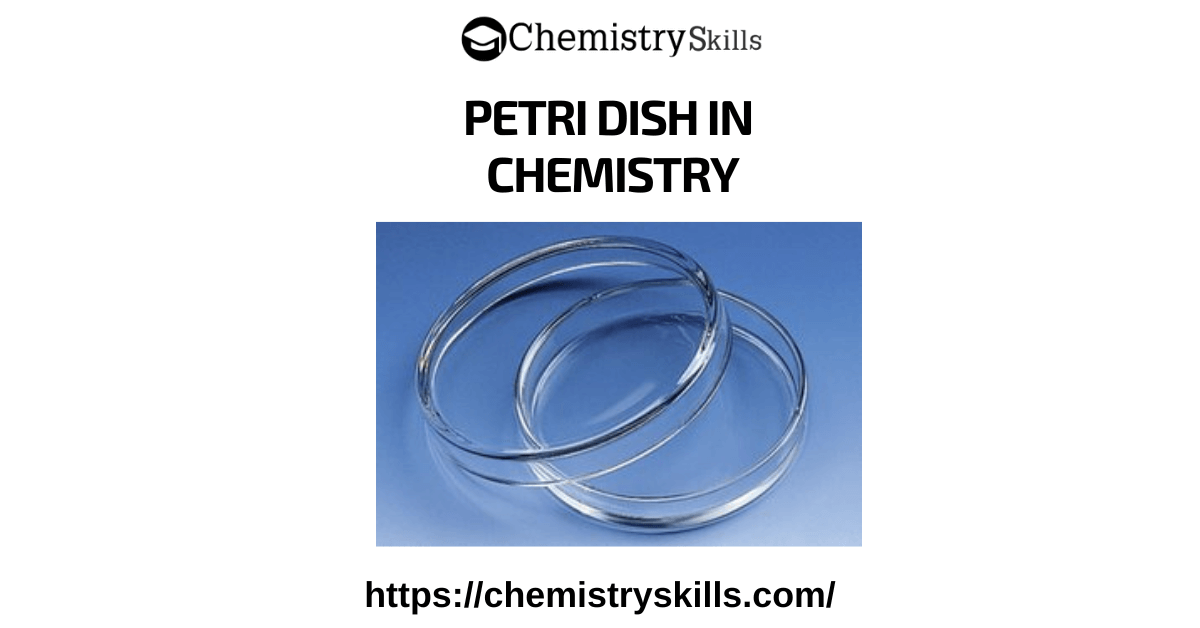
In the quantum mechanical description of the atom, electrons’ motion can only be represented using the concept of probability. The orbitals traveled by electrons are not identifiable as trajectories but as ‘electronic clouds’ whose density is proportional to the probability of finding the electron in that region.
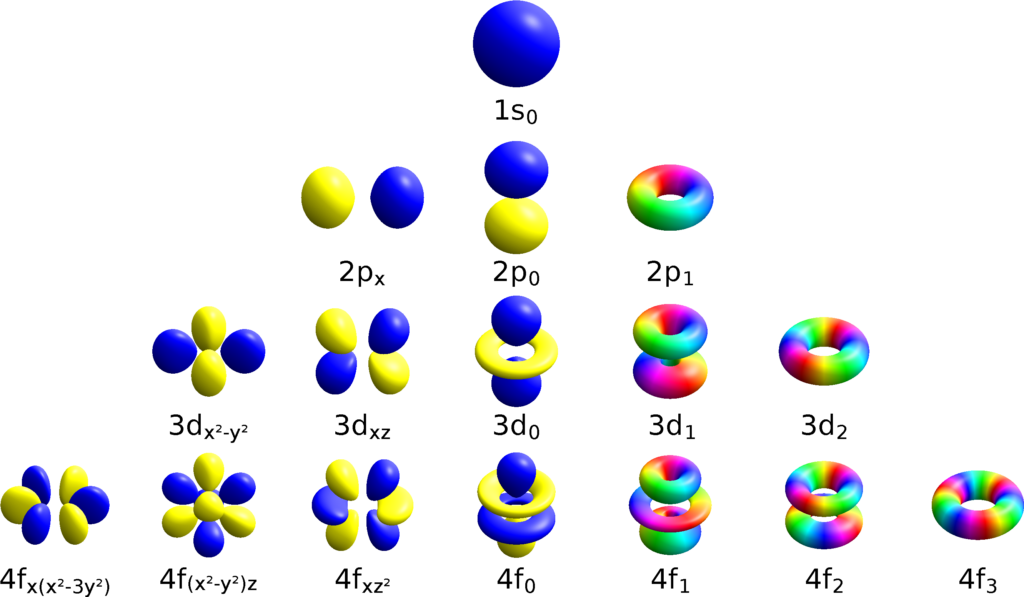
In the wave function ψ appear some numerical constants called quantum numbers
• Each orbital is uniquely determined by three quantum numbers n, l, and m.
• Each orbital can hold a maximum of 2 electrons, which differ by a fourth quantum number s (spin).
• The state of an electron in an atom is defined by its four quantum numbers n, l, m, ms.
Each atomic orbital has a unique set of these quantum numbers.
Principal Quantum Number (n)
Principal quantum number determines the size of the orbital. The larger n is the greater the distance from the nucleus. n = 1,2,3 …7, it corresponds to the quantum number of the Bohr model; in that theory, n automatically determined the orbit’s energy content, but this rule is no longer valid for a ‘multielectronic’ atom.
Azimuthal or Angular Momentum (l)
Describes the shape of atomic orbitals. The values of l are integers and depend on the value of the principal quantum number n.
One of the letters s, p, d, and f is associated with each value of l.
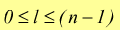
| l | 0 | 1 | 2 | 3 |
| s | p | d | f |
- A set of orbitals having the same value of n is called a level.
- One or more orbitals having the same value of n and l are called sublevel.
E.g., level n = 2 is composed of two sublevels l = 0 and l = 1. These sublevels are called 2s and 2p, where 2 represents the value of n and s & p the value of l.
Magnetic Quantum Number (m)
Describes the orientation of orbitals in space. Within a sublevel, the value of m depends on the value of l. For some value of l, there are 2l+1 integer values of m.
If l = 1 there are three possible values of m: -1.0, + 1.
For l = 2 the possible values are five m: -2, -1.0, + 1, + 2.
For l = 3 the possible values are seven m: -3, -2, -1, 0, + 1, + 2. + 3 and so on.
The number of values of m indicates the number of orbitals in a sublevel with a particular value of l; each value of m refers to a different orbital.
The total number of values of m coincides with the total number of possible orbitals for a given value of n.
So given [n], there are n2 orbitals and for a maximum of 2n2 electrons.
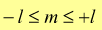
Spin Quantum Number (ms)
Schrödinger’s calculation of hydrogen orbitals’ energy constituted a milestone in the development of modern atomic theory; however, the spectral lines did not show precisely the frequency he predicted. In 1925 (before Schrödinger’s work but after Bohr’s first atomic model), two American physicists of Dutch origin, Samuel Goudsmit, and George Uhlenbeck proposed the explanation for the slight differences observed. They suggested that an electron behaves in some ways like a spinning sphere, something like a planet spinning around its axis. This property constitutes the so-called spin.
The electron has access to two spin states, represented with the arrows ↑ and ↓ or with the Greek letters α and β. We can imagine that the electron can rotate counterclockwise at a certain speed (state ↑) or clockwise at precisely the same speed (state ↓). The two spin states are characterized by a fourth quantum number, the magnetic spin quantum number ms.
From these observations, we deduce:
• An s orbital contains a maximum of 2 electrons
• A p orbital contains a maximum of 6 electrons
• A d orbital contains a maximum of 10 electrons
• An f orbital contains a maximum of 14 electrons